N1CTF 2022 Crypto Writeups By tl2cents

2022-11-08

字数统计: 2.7k字 | 阅读时长≈ 16分

In this competition, NeSE solved all the reversing , misc , crypto challenges and won the championship. Here are the detailed writeups of three crypto challenges.

brandnew check-in

Source Code

from Crypto.Util.number import *
from random import getrandbits
from secret import flag

def keygen():
    p = getPrime(512)
    q = getPrime(512)
    n = p * q
    phi = (p-1)*(q-1)
    while True:
        a = getrandbits(1024)
        b = phi + 1 - a
        s = getrandbits(1024)
        t = -s*a * inverse(b, phi) % phi
        if GCD(b, phi) == 1:
            break
    return (s, t, n), (a, b, n)

def enc(m, k):
    s, t, n = k
    r = getrandbits(1024)
    return m * pow(r, s, n) % n, m * pow(r, t, n) % n

pubkey, privkey = keygen()
flag = pow(bytes_to_long(flag), 0x10001, pubkey[2])
c = []
for m in long_to_bytes(flag):
    c1, c2 = enc(m, pubkey)
    c.append((c1, c2))
print(pubkey)
print(c)

Solution

By the description : cakectf brand_new_crypto, I went for Google to browse the writeup and found this one : CakeCTF 2022 Writeup. We can use the extended Euclid algorithm to obtain x,y such that : $s*x+t*y = 1$ . Therefore, $c_1^x*c_2^y = m^{x+y}*r^{s*x+t*y}=m^{x+y}*r$ , we can brute force $m \in [0,255]$ to recover the $r_0$ and use the $r_0$ to verify the original equations : $c_1 = m*r^s,\ c_2=m*r^t$ . By this method, we can recover all the $m_i$ and $r_i$ if $m\ne0$ .

Now, we have recovered the encrypted flag : $encflag = pow(flag,e,n)$ . Notice that $r_i$ is generated with getrandbits(1024), we can fully recover MT19937 state and then backtrack to find parameters $a$ or even $p,q$ . Here, parameter $a$ is easy to locate because it is exactly the previous 1024 bits of $s$ . The most important point of recovering MT state is that our recovered $r_i = getrandbits(1024) \mod n$ . Therefore, each $r_i$ has 2~3 possible values and we need 20 consecutive parameters $r_i,..,r_{i+19}$ to reconstruct MT state and another 20 consecutive parameters $r_{i+20},..,r_{i+39}$ to verify (if not verified, there might be multiple solutions). I found the minimal brute-forcing space is $2^{23}$ .

After we recovered $a$ , $t*b = -sa \mod \varphi(n) \iff t*(1-a) + s*a = 0 \mod \varphi(n)$ . Therefore, we can obtain $k*\varphi(x)$ and derive the private key to decrypt $encflag$ .

EXP

from ast import literal_eval
from Crypto.Util.number import *
from tqdm import tqdm

lines = open("./output.txt","r").readlines()
s,t,n = literal_eval(lines[0].strip())
enc = literal_eval(lines[1])

def xgcd(a: int, b: int):
    x0, y0, x1, y1 = 0, 1, 1, 0
    while a != 0:
        q, a, b = b // a, b % a, a
        x0, x1 = x1, x0 - x1 * q
        y0, y1 = y1, y0 - y1 * q
    return b, x0, y0

encflag = b""
r_list = []

for c1, c2 in tqdm(enc):
    if c1 == 0 and c2 == 0:
        # m is zero , r unknown, set -1
        encflag += bytes([0])
        r_list.append(-1)
        continue
    for m in range(256):
        g, x, y = xgcd(s, t)
        z = pow(c1, x, n)
        w = pow(c2, y, n)
        mm = pow(m, -(x+y), n)
        r_ = mm * z * w % n
        c1_ = (m * pow(r_, s // g, n)) % n
        c2_ = (m * pow(r_, t // g, n)) % n
        if c1_ == c1 and c2_ == c2:
            encflag += bytes([m])
            r_list.append(r_)
            break
print(encflag)

MT Cracker (offset = 48, the brute-forcing space is minimal, 12 cores multi-process, time cost : 6 minutes):

# Initialise rng (random.getrandbits)
import random
import time
import copy
from sympy import prod
from Crypto.Util.number import *
from ast import literal_eval
from tqdm import tqdm
from mt19937predictor import MT19937Predictor

off = 48
lines = open("./output.txt","r").readlines()
s,t,n = literal_eval(lines[0].strip())
enc = literal_eval(lines[1])

r = r_list # above r_list

data = r[off:20+off] 
multi_r_list = []

def split32bit(d):
    temp = []
    for _ in range(1024//32):
        temp.append(d%2**32)
        d = d>>32
    assert d==0
    return temp
    
for d in data[:20]:
    assert d < n
    multi_ls = [split32bit(d)]
    while d + n < 2**1024:
        d = d + n
        multi_ls.append(split32bit(d))
    multi_r_list.append(multi_ls)
    
from itertools import product
brute_length = prod([len(i) for i in multi_r_list])
print(f"[+] Brute forcing space {brute_length} {brute_length.bit_length()}")

# split the brute-forcing space
expand_part = product(*(multi_r_list[-3:]))
brute_list = [product(*(multi_r_list[:-3]+[[f[0]],[f[1]],[f[2]]])) for f in expand_part]
n_cores = len(brute_list)
slice_length = brute_length//n_cores
datas = [[b] for b in brute_list]
print(f"[+] cores {n_cores}")

def verify_func(brute_list):
    for i in tqdm(brute_list,total=slice_length):
        MT_data = []
        for ls in i:
            MT_data.extend(ls)
        predictor = MT19937Predictor()
        for _ in range(624):
            predictor.setrandbits(MT_data[_], 32)
            
        mflag = True
        for _ in range(640-624):
            if predictor.getrandbits(32)!= MT_data[624 + _]:
                mflag = False
                break
        if mflag == False:
            continue
        # print(f"[+] possible result : {MT_data}")
        for _ in range(20):
            if predictor.getrandbits(1024)%n != r[20+off+_]:
                mflag = False
                break
        if mflag == False:
            continue
        print(f"[+] GOOD MT STATE")
        return (True,MT_data[:])
    return (False,[])
    
from tqdm import tqdm
from concurrent.futures import ProcessPoolExecutor, as_completed
import multiprocessing
import os


def parallel_process(array, function, use_kwargs=False):
    """
        A parallel version of the map function with a progress bar. 

        Args:
            array (array-like): An array to iterate over.
            function (function): A python function to apply to the elements of array
            n_jobs (int, default=16): The number of cores to use
            use_kwargs (boolean, default=False): Whether to consider the elements of array as dictionaries of 
                keyword arguments to function 
            front_num (int, default=3): The number of iterations to run serially before kicking off the parallel job. 
                Useful for catching bugs
        Returns:
            [function(array[0]), function(array[1]), ...]
    """
    front = []
    n_jobs = n_cores
    with ProcessPoolExecutor(max_workers=n_jobs) as pool:
        if use_kwargs:
            futures = [pool.submit(function, **a) for a in array]
        else:
            futures = [pool.submit(function, *a) for a in array]
        kwargs = {
            'total': len(futures),
            'unit': 'it',
            'unit_scale': True,
            'leave': True
        }
        #Print out the progress as tasks complete
        for f in tqdm(as_completed(futures), **kwargs):
            res = f.result()
            if res[0] == True:
                print("[+] find!")
                print(res)
                pool.shutdown(wait=True, cancel_futures=True)
                break
    return res

res_list = parallel_process(datas,verify_func)

[+] Brute forcing space 7962624 23
[+] cores 12
 69%|██████████████████████████████████████████████████▍                      | 458676/663552 [07:08<02:57, 1153.05it/s]
[+] GOOD MT STATE

data = res_list # results from above MT state
import random
from Crypto.Util.number import *
from ast import literal_eval
from tqdm import tqdm

lines = open("./output.txt","r").readlines()
s,t,n = literal_eval(lines[0].strip())
enc = literal_eval(lines[1])

# https://github.com/JuliaPoo/MT19937-Symbolic-Execution-and-Solver
import sys
sys.path.append('./source')
# Import symbolic execution
from MT19937 import MT19937, MT19937_symbolic
# Import XorSolver
from XorSolver import XorSolver
rng = lambda: random.getrandbits(1024)

rng_clone = MT19937(state_from_data = (data, 32))
shift = 64*(1024//32)
rng_clone.reverse_states(shift)

def get1024():
    res = 0 
    temps = []
    for i in range(32):
        temps.append(rng_clone())
    for num in temps[::-1]:
        res = (res<<32) + num
    return res


res_ls = []
for _ in range(64):
    rnd_num = get1024()
    res_ls.append(rnd_num)
    if rnd_num%n == s%n:
        print("[+] cracked")
        a = res_ls[-2]
        break
        
kphi = s*a+(1-a)*t
print(f"[+] kphi found , verify {pow(2,kphi,n) == 1} ")
encflag = b'\x08EZg\xbf\xa0\xeb\x9d\x81\x01\xa8\x96m\x97\x08I(\xed\xb5iQE\xdb\xf5\x8c\xbdcr!\xe6\xc9\xac\x0c\x16K\xa0\x0fr\xecM\x04\xe6\x87\x0f}9\x94\xcfa\x16\x87\x8f4\xcd\xcb\xa4\x0eq\xc3Q\x16\x928&\xe2\x18C\xafN\x87\xcc\x18\xc2D\x9d\x06\xbd"\xe7\xe8\xb7\x12\xb0\xb8CC\x9aM\xff\x12\x00\x05,\xeeopYC)mI\xb7\x81\xb6\x13\x0e\x8a\xc0\xd7\xd3\xd2\xa9\xe5vg.\xa4\xf3\xaa\x10f\x9c\xa4nS=O\xe9'
encnum = bytes_to_long(encflag)
d = inverse(0x10001,kphi)
m = long_to_bytes(pow(encnum,d,n))
print(m)

ezdlp

Source Code

from Crypto.Util.number import *
from math import prod
from secret import flag

def keygen(pbits,kbits,k):
    p = getPrime(pbits)
    x = [getPrime(kbits + 1) for i in range(k)]
    y = prod(x)
    while 1:
        r = getPrime(pbits - kbits * k)
        q = 2 * y * r + 1
        if isPrime(q):
            return p*q, (p, q, r, x)

def encrypt(key, message):
    return pow(0x10001, message, key)

key = keygen(512, 24, 20)
flag = bytes_to_long(flag)
messages = [getPrime(flag.bit_length()) for i in range(47)] + [flag]
enc = [encrypt(key[0], message) for message in messages]

print(messages[:-1])
print(enc)

Solution

Use lattice and gcd to recover the correct modulo $N$ . Since $N$ has a semi-smooth order prime ( $B_1 = 2^{25}, B_2 = 2^{32}$ ) , we can use Pollard p-1 algorithm to factorize $N$ and Pohlig-Hellman algorithm to compute the discrete logarithm to obtain flag.

Recover modulo N

The first step to recover the modulo N. Since the exponent is too large to be computed in Integer Ring, we can construct a lattice to narrow down the exponents. The lattice is as follows:

$M = \begin{bmatrix} m_1 & 1 & 0 &... & 0 \\ m_2 & 0 & 1 &... & 0 \\ ... & ... & ... & ... & ... \\ m_{47} & 0 & 0 &... & 1 \\ \end{bmatrix} \tag{1}$

Apply LLL algorithm to M: $L = M.LLL()$ . Denoting $L_i = [r_{i},k_{i1},...,k_{i47}]$ as the ith row of $L$ , we have the following equations :

$\sum\limits_{j=1}^{47} m_j*k_{ij} = r_i \tag{2}, \quad i = 1,2,..,47$

In (2), if $k_{ij} < 0$ , place it on the left side; if $r_i<0$ , place it on the right side. Now every parameter is positive, and we exponentiate both sides of the equation with base = 0x10001 and then subtract them to get integer multiples of modulo $N$ . We can compute $n = gcd(kn_1,kn_2,..,kn_{47})$ and then filter all the small factors to recover the real modulo $N$ (about 1034 bits).

Semi-Smooth Factorization and Discrete Logarithm

If we simply run Pollard’s p-1 algorithm on $N$ , it costs about 10 hours in sage with time complexity $2^{32}$ . This TCC paper explains the concept of semi-smooth and related factorization attacks. Yafu implements such factorization. Assume the two bounds for semi-smooth order prime are : $B_1 = 2^a,\ B_2 = 2^b , b>a$ , the time complexity is $O(2^a +\sqrt{2^b})$ . In the challenge, the time complexity is $O(2^{25})$ .

For yafu, the max $B_1$ bound is $2^{32}$ and for $B_2$ , the upper bound should be $2^{64}$ . If you find that with correct $B1,B_2$ you can’t obtain the factorization, you can increase the bound $B_2$ a little and run again.

The yafu documentation of pm1:

[pm1]
usage: pm1(expression)

description:
New in version 1.28: uses GMP-ECM exclusively. Stage 1 bound is configurable using
the POLLARD_STG1_MAX parameter. The default is 100000. Stage 2 bound is also
configurable using the POLLARD_STG2_MAX parameter.

To use the default B2 with gmp-ecm, simply do not specify the B2ecm or B2pp1 or B2pm1
flags in the .ini file or in the command line arguments.
Specifying B2 as well will cause the default value to be overridden.

command line flags affecting pm1:

-B1pm1 B1 bound in the pm1 method
-B2pm1 B2 bound in the pm1 method

Crack our modulo in this challenge, a few seconds:

C:\yafu-1.34>yafu-x64.exe -B1pm1 33554432 -B2pm1 4294967296

11/07/22 14:55:23 v1.34.5 @ TL2CENTS, System/Build Info:
Using GMP-ECM 6.3, Powered by GMP 5.1.1
detected 12th Gen Intel(R) Core(TM) i7-12700H
detected L1 = 49152 bytes, L2 = 25165824 bytes, CL = 64 bytes
measured cpu frequency ~= 2692.260400
using 20 random witnesses for Rabin-Miller PRP checks

===============================================================
======= Welcome to YAFU (Yet Another Factoring Utility) =======
=======             bbuhrow@gmail.com                   =======
=======     Type help at any time, or quit to quit      =======
===============================================================
cached 78498 primes. pmax = 999983


>> pm1(131158523227880830085100826212925738665356578827561846263073537503153187073136528966506785633847097997799377037969243883439723340886038624250936927221630287086602285835045356221763554989140952262353930420392663280482277832613695689454662506372252641564106136178637816827646124189347219273164844809807934422046441)

pm1: starting B1 = 33554432, B2 = 4294967296 on C312


***factors found***

P158 = 12980311456459934558628309999285260982188754011593109633858685687007370476504059552729490523256867881534711749584157463076269599380216374688443704196597025947

***co-factor***
P155 = 10104420349837363561278745998119091841853342383118385156657416134976061697027571349895988817770681767227605656666215380267313369652920490697343475330713803

ans = 10104420349837363561278745998119091841853342383118385156657416134976061697027571349895988817770681767227605656666215380267313369652920490697343475330713803

After factorization, just use discrete_log of sage to obtain the flag.

EXP

Lattice to compute $k*n$

from ast import literal_eval

lines = open("./output.txt","r").readlines()
messages = literal_eval(lines[0].strip())
enc = literal_eval(lines[1].strip())

M1 = matrix.identity(ZZ,47)
B1 = matrix(ZZ,47,1) 
for i,message in enumerate(messages):
    B1[i,0] = message

M = block_matrix(ZZ,[B1,M1],ncols = 2)
L = M.LLL()

def coompute_kn(coff):
    # \sum mi*ki = coff[0]
    if coff[0]>0:
        res_right = 1
        res_left = pow(0x10001, coff[0])
    else:
        res_right = pow(0x10001, -coff[0])
        res_left = 1
    for i ,cof in enumerate(coff[1:]):
        if cof > 0:
            res_right = res_right * enc[i]**cof
        else:
            res_left = res_left * enc[i]**(-cof)
    return res_left - res_right

n0 = coompute_kn(L[0])
for i in range(1,47):
    kn = coompute_kn(L[i])
    n0 = gcd(kn,n0)
    print("[+] nbits ",n0.nbits())
    if n0.nbits()<=1024:
        print(n0)
        break
print(n0)
# filter small factors
n0 = factor(n0,limit=2^20)
n_ = n0[-1][0]
print(f"[+] find n : {n_}")
print(f"[+] n bits : {n_.nbits()}")

Factorization by yafu , then compute discrete logarithm:

from Crypto.Util.number import *
encm = enc[-1]
# discrete_log
p = 12980311456459934558628309999285260982188754011593109633858685687007370476504059552729490523256867881534711749584157463076269599380216374688443704196597025947
q = 10104420349837363561278745998119091841853342383118385156657416134976061697027571349895988817770681767227605656666215380267313369652920490697343475330713803
assert p*q==n_
GN = GF(p)
flag = discrete_log(GN(encm),GN(0x10001))
print(long_to_bytes(int(flag)))

babyecc

Source Code

from Crypto.Util.number import *
from secret import flag

m = Integer(int.from_bytes(flag, 'big'))

for _ in range(7):
    p = getPrime(512)
    q = getPrime(512)
    n = p * q
    while 1:
        try:
            a = randint(0,n)
            b = randint(0,n)
            Ep = EllipticCurve(GF(p), [a,b])
            Gp = Ep.lift_x(m) * 2
            Eq = EllipticCurve(GF(q), [a,b])
            Gq = Eq.lift_x(m) * 2
            y = crt([int(Gp[1]),int(Gq[1])],[p,q])
            break
        except Exception as err:
            pass
    print(n, a, b, y)

Solution

First use the point doubling formula to construct an equation ( $g(m,a,b,y) = 0 \mod n$ ) for every set of $(n,a,b,y)$ . Combine these polynomials $f_i(m) = g(m,a_i,b_i,y_i)$ with CRT to derive a new polynomial $F(m) \mod \prod n_i$ . Finally we can get the small root of $F$ (coppersmith method) and recover the flag.

Construct Polynomial

Point $(x,y)$ doubling formula of Weierstrass normal form ECC :

$\left\{ \begin{array}{lr} y^2 = x^3 +ax+b\\ \lambda = \frac{3x^2+1}{2y} \\ x_r = \lambda^2 -2x \\ y_r = \lambda (x-x_r) -y \\ \end{array} \right. \tag{1}$

Consider the double point with x-coordinate= $m$ , from (1), $x_r = (\frac{3m^2+a}{2y})^2-2m = \frac{(3m^2+a)^2}{4(m^3+am+b)}-2m$ , multiply both sides with $4(m^3+am+b)$ :

$4(m^3+am+b)x_r = (3m^2+a)^2-8m(m^3+am+b) \tag{2}$

The ECC polynomial :

$y_r^2 = x_r^3 +ax + b \tag{3}$

Denote k, c as :

$\left\{ \begin{array}{lr} k =4(m^3+am+b)\\ c = (3m^2+a) \\ \end{array} \right.$

Combine (2), (3) :

$y_r^2 = (\frac{c}{k}-2m)^3 +a(\frac{c}{k}-2m) + b \implies k^3 y_r^2 -[(c-2mk)^3 +a(k^2c-2mk^3)+bk^3] = 0 \tag{4}$

Now we have constructed a polynomial $f(x)$ modulo $n$ and $f(m)=0$ .

SMUPE-problem

In this section, we focus on solving Systems of Modular Univariate Polynomial Equations (SMUPE).

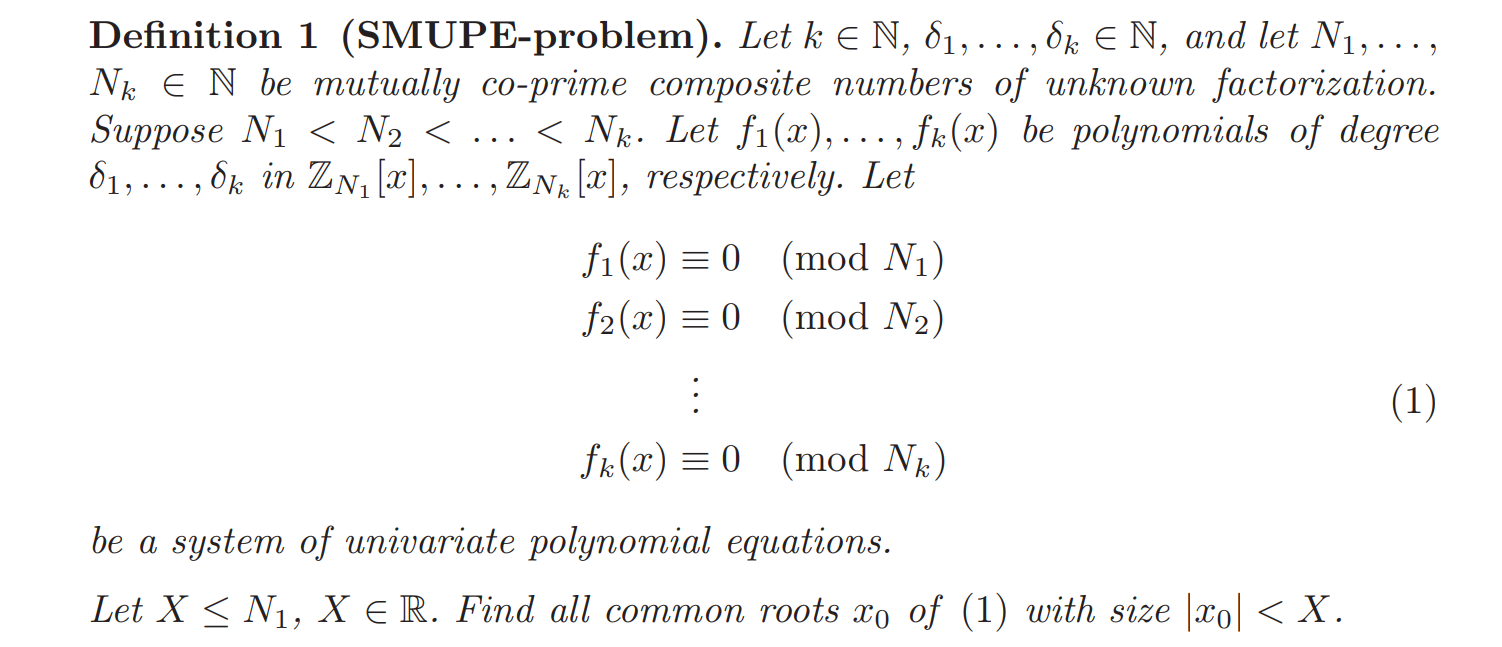
SMUPE

A straight forward method : combine all the polynomials with CRT and we can obtain a new polynomial $F(x) \mod \prod n_i$ which coppersmith method can be applied to. Let the max degree of $f_i$ is $\theta$ and every $N_i$ is about $2^{nbit}$ , the upper bound of common root $x_o$ is $2^{k\times nbits/ \theta}$ . In this challenge, we have 7 polynomials; the degree of all $f_i$ is 12; the bits of $N_i$ is 1024. Therefore, the upper bound of $x_0$ for the above idea is $2^{7*1024/12} \approx 2^{597}$ . Our flag length is 344 bits. F(x).small_roots(X=2^400,epsilon= 1/40,beta = 4/7) is enough to extract the small root $m$ .

EXP

from ast import literal_eval
lines = open("./output.txt","r").readlines()
fs = []
ns = []
para_s = []

def fun(n,a,b,y):
    Pn.<x> = PolynomialRing(Zmod(n))
    k = 4*(x^3+a*x+b)
    c = (3*x^2+a)^2
    f = k^3*y^2 - (c-2*x*k)^3 - a*(k^2*c-2*x*k^3) - b*k^3
    return f

for line in lines:
    n,a,b,y = [ZZ(i) for i in line.strip().split(" ")]
    f = fun(n,a,b,y).monic().change_ring(ZZ)
    fs.append(f)
    ns.append(n)
    para_s.append([n, a, b, y])
    
F = crt(fs,ns)
M = prod(ns)
FF = F.change_ring(Zmod(M))
roots = FF.small_roots(X = 2**400,epsilon = 1/40,beta = 4/7)
# m = FF.small_roots(X=2**256,beta=1.0)
print(roots)

from Crypto.Util.number import *
m = 15425251357776807541227287240467548867067510789583043568768907891381811730130189461693802496389917454461
print(m.nbits())
print(long_to_bytes(int(m)))